0 attempts
0% avg
UBQ Credits
Pro Tip – Draw an FBD to visualize the all forces and lever arms acting on the ladder. Note that you can split either the forces or the lever arm into components as long as the two are are perpendicular to each other.
| Step | Derivation/Formula | Reasoning |
|---|---|---|
| 1 | [katex] h = L \sin(\theta) [/katex] | Calculate the height [katex] h [/katex] of the ladder against the wall using the sine function where [katex] \theta [/katex] is the angle with the ground. |
| 2 | [katex] h = 5 \sin(60^\circ) = 5 \times \frac{\sqrt{3}}{2} \approx 4.33 \, \text{m} [/katex] | The angle [katex] \theta [/katex] is given as [katex] 60^\circ [/katex]. The [katex] \sin(60^\circ) = \frac{\sqrt{3}}{2} [/katex]. |
| 3 | [katex] w_{\text{lad}} = mg [/katex] [katex] w_{\text{lad}} = 20 \times 9.8 = 196 \, \text{N} [/katex] |
Calculate the weight of the ladder using its mass [katex] m [/katex] and gravitational acceleration [katex] g [/katex]. |
| 4 | [katex] w_{\text{person}} = m_{\text{person}}g [/katex] [katex] w_{\text{person}} = 80 \times 9.8 = 784 \, \text{N} [/katex] |
Calculate the weight of the person using the person’s mass [katex] m_{\text{person}} [/katex] and gravitational acceleration [katex] g [/katex]. |
| 5 | [katex] \text{Moment at the bottom} = \text{Moment at the top} [/katex] | The torque or moment due to the person and the ladder about the point where the bottom of the ladder contacts the ground must be balanced by the force exerted by the wall. |
| 6 | [katex] F_{\text{wall}} \times h = w_{\text{lad}} \times \frac{L}{2} \cos(\theta) + w_{\text{person}} \times d \cos(\theta) [/katex] | The moment (or torque) at the top due to the force from the wall [katex] F_{\text{wall}} [/katex] must counterbalance the moments generated by the weight of the ladder and person. [katex] L [/katex] is the ladder length, [katex] d [/katex] is the distance where the person stands from the bottom. |
| 7 | [katex] F_{\text{wall}} \times 4.33 = 196 \times \frac{5}{2} \times \frac{1}{2} + 784 \times 4 \times \frac{1}{2} [/katex] | Substitute values for [katex] L = 5 \, \text{m}, d = 4 \, \text{m}, \cos(60^\circ) = \frac{1}{2}, h \approx 4.33 \, \text{m} [/katex]. |
| 8 | [katex] F_{\text{wall}} \times 4.33 = 98 \times 2.5 + 784 \times 2 [/katex] | Simplification of the equation to compute the force exerted by the wall. |
| 9 | [katex] F_{\text{wall}} \times 4.33 = 245 + 1568 [/katex] | Total moments at the top due to the weight of both the ladder and person. |
| 10 | [katex] F_{\text{wall}} \times 4.33 = 1813 [/katex] | Add the moments for the final calculation. |
| 11 | [katex] F_{\text{wall}} = \frac{1813}{4.33} \approx 418.71 \, \text{N} [/katex] | Calculate the force exerted by the wall by dividing the total moment by the height [katex] h [/katex]. |
| 12 | [katex] F_{\text{wall}} \approx 419 \, \text{N} [/katex] | Finding the final value and rounding off to the nearest whole number, providing the force in Newtons. |
Just ask: "Help me solve this problem."
An \( 80 \, \text{kg} \) block is placed \( 2 \, \text{m} \) away from the endpoint of a horizontal steel beam of length \( 6.6 \, \text{m} \) and mass \( 1,450 \, \text{kg} \). The plank makes contact with a vertical wall on one end, and the other endpoint is attached to a massless cable that makes an angle of \( 30^\circ \) with the horizontal and ties into the vertical wall as well. Calculate the magnitude and direction of the force between the cable and the wall and of the force between the steel beam and the wall.
The diagram above shows a hydraulic chamber with a spring \( (k_s = 1250 \, \text{N/m}) \) attached to the input piston and a rock of mass \( 55.2 \, \text{kg} \) resting on the output plunger. The input piston and output plunger are at about the same height, and each has negligible mass. The chamber is filled with water.
The diagram above shows a top view of a child of mass \(M\) on a circular platform of mass \(2M\) that is rotating counterclockwise. Assume the platform rotates without friction. Which of the following describes an action by the child that will increase the angular speed of the platform-child system and gives the correct reason why?
A meter stick of mass [katex] .2 [/katex] kg is pivoted at one end and supported horizontally. A force of [katex] 3 [/katex] N downwards is applied to the free end, perpendicular to the length of the meter stick. What is the net torque about the pivot point?
A car is moving up the side of a circular roller coaster loop of radius \( 12 \) \( \text{m} \). The angular velocity is \( 1.8 \) \( \text{rad/s} \) and angular acceleration is \( -0.82 \) \( \text{rad/s}^2 \). The car is at the same elevation as the center of the loop. Find the magnitude and direction (relative to the horizontal) of the acceleration.
419 N
By continuing you (1) agree to our Terms of Sale and Terms of Use and (2) consent to sharing your IP and browser information used by this site’s security protocols as outlined in our Privacy Policy.
| Kinematics | Forces |
|---|---|
| \(\Delta x = v_i t + \frac{1}{2} at^2\) | \(F = ma\) |
| \(v = v_i + at\) | \(F_g = \frac{G m_1 m_2}{r^2}\) |
| \(v^2 = v_i^2 + 2a \Delta x\) | \(f = \mu N\) |
| \(\Delta x = \frac{v_i + v}{2} t\) | \(F_s =-kx\) |
| \(v^2 = v_f^2 \,-\, 2a \Delta x\) | Â |
| Circular Motion | Energy |
|---|---|
| \(F_c = \frac{mv^2}{r}\) | \(KE = \frac{1}{2} mv^2\) |
| \(a_c = \frac{v^2}{r}\) | \(PE = mgh\) |
| \(T = 2\pi \sqrt{\frac{r}{g}}\) | \(KE_i + PE_i = KE_f + PE_f\) |
| Â | \(W = Fd \cos\theta\) |
| Momentum | Torque and Rotations |
|---|---|
| \(p = mv\) | \(\tau = r \cdot F \cdot \sin(\theta)\) |
| \(J = \Delta p\) | \(I = \sum mr^2\) |
| \(p_i = p_f\) | \(L = I \cdot \omega\) |
| Simple Harmonic Motion | Fluids |
|---|---|
| \(F = -kx\) | \(P = \frac{F}{A}\) |
| \(T = 2\pi \sqrt{\frac{l}{g}}\) | \(P_{\text{total}} = P_{\text{atm}} + \rho gh\) |
| \(T = 2\pi \sqrt{\frac{m}{k}}\) | \(Q = Av\) |
| \(x(t) = A \cos(\omega t + \phi)\) | \(F_b = \rho V g\) |
| \(a = -\omega^2 x\) | \(A_1v_1 = A_2v_2\) |
| Constant | Description |
|---|---|
| [katex]g[/katex] | Acceleration due to gravity, typically [katex]9.8 , \text{m/s}^2[/katex] on Earth’s surface |
| [katex]G[/katex] | Universal Gravitational Constant, [katex]6.674 \times 10^{-11} , \text{N} \cdot \text{m}^2/\text{kg}^2[/katex] |
| [katex]\mu_k[/katex] and [katex]\mu_s[/katex] | Coefficients of kinetic ([katex]\mu_k[/katex]) and static ([katex]\mu_s[/katex]) friction, dimensionless. Static friction ([katex]\mu_s[/katex]) is usually greater than kinetic friction ([katex]\mu_k[/katex]) as it resists the start of motion. |
| [katex]k[/katex] | Spring constant, in [katex]\text{N/m}[/katex] |
| [katex] M_E = 5.972 \times 10^{24} , \text{kg} [/katex] | Mass of the Earth |
| [katex] M_M = 7.348 \times 10^{22} , \text{kg} [/katex] | Mass of the Moon |
| [katex] M_M = 1.989 \times 10^{30} , \text{kg} [/katex] | Mass of the Sun |
| Variable | SI Unit |
|---|---|
| [katex]s[/katex] (Displacement) | [katex]\text{meters (m)}[/katex] |
| [katex]v[/katex] (Velocity) | [katex]\text{meters per second (m/s)}[/katex] |
| [katex]a[/katex] (Acceleration) | [katex]\text{meters per second squared (m/s}^2\text{)}[/katex] |
| [katex]t[/katex] (Time) | [katex]\text{seconds (s)}[/katex] |
| [katex]m[/katex] (Mass) | [katex]\text{kilograms (kg)}[/katex] |
| Variable | Derived SI Unit |
|---|---|
| [katex]F[/katex] (Force) | [katex]\text{newtons (N)}[/katex] |
| [katex]E[/katex], [katex]PE[/katex], [katex]KE[/katex] (Energy, Potential Energy, Kinetic Energy) | [katex]\text{joules (J)}[/katex] |
| [katex]P[/katex] (Power) | [katex]\text{watts (W)}[/katex] |
| [katex]p[/katex] (Momentum) | [katex]\text{kilogram meters per second (kgm/s)}[/katex] |
| [katex]\omega[/katex] (Angular Velocity) | [katex]\text{radians per second (rad/s)}[/katex] |
| [katex]\tau[/katex] (Torque) | [katex]\text{newton meters (Nm)}[/katex] |
| [katex]I[/katex] (Moment of Inertia) | [katex]\text{kilogram meter squared (kgm}^2\text{)}[/katex] |
| [katex]f[/katex] (Frequency) | [katex]\text{hertz (Hz)}[/katex] |
General Metric Conversion Chart
Example of using unit analysis: Convert 5 kilometers to millimeters.Â
Start with the given measurement: [katex]\text{5 km}[/katex]
Use the conversion factors for kilometers to meters and meters to millimeters: [katex]\text{5 km} \times \frac{10^3 \, \text{m}}{1 \, \text{km}} \times \frac{10^3 \, \text{mm}}{1 \, \text{m}}[/katex]
Perform the multiplication: [katex]\text{5 km} \times \frac{10^3 \, \text{m}}{1 \, \text{km}} \times \frac{10^3 \, \text{mm}}{1 \, \text{m}} = 5 \times 10^3 \times 10^3 \, \text{mm}[/katex]
Simplify to get the final answer: [katex]\boxed{5 \times 10^6 \, \text{mm}}[/katex]
Prefix | Symbol | Power of Ten | Equivalent |
|---|---|---|---|
Pico- | p | [katex]10^{-12}[/katex] | |
Nano- | n | [katex]10^{-9}[/katex] | |
Micro- | µ | [katex]10^{-6}[/katex] | |
Milli- | m | [katex]10^{-3}[/katex] | |
Centi- | c | [katex]10^{-2}[/katex] | |
Deci- | d | [katex]10^{-1}[/katex] | |
(Base unit) | – | [katex]10^{0}[/katex] | |
Deca- or Deka- | da | [katex]10^{1}[/katex] | |
Hecto- | h | [katex]10^{2}[/katex] | |
Kilo- | k | [katex]10^{3}[/katex] | |
Mega- | M | [katex]10^{6}[/katex] | |
Giga- | G | [katex]10^{9}[/katex] | |
Tera- | T | [katex]10^{12}[/katex] |
The most advanced version of Phy. 50% off, for early supporters. Prices increase soon.
per month
Billed Monthly. Cancel Anytime.
Trial –> Phy Pro
Try our free calculator to see what you need to get a 5 on the upcoming AP Physics 1 exam.
Understand you mistakes quicker.
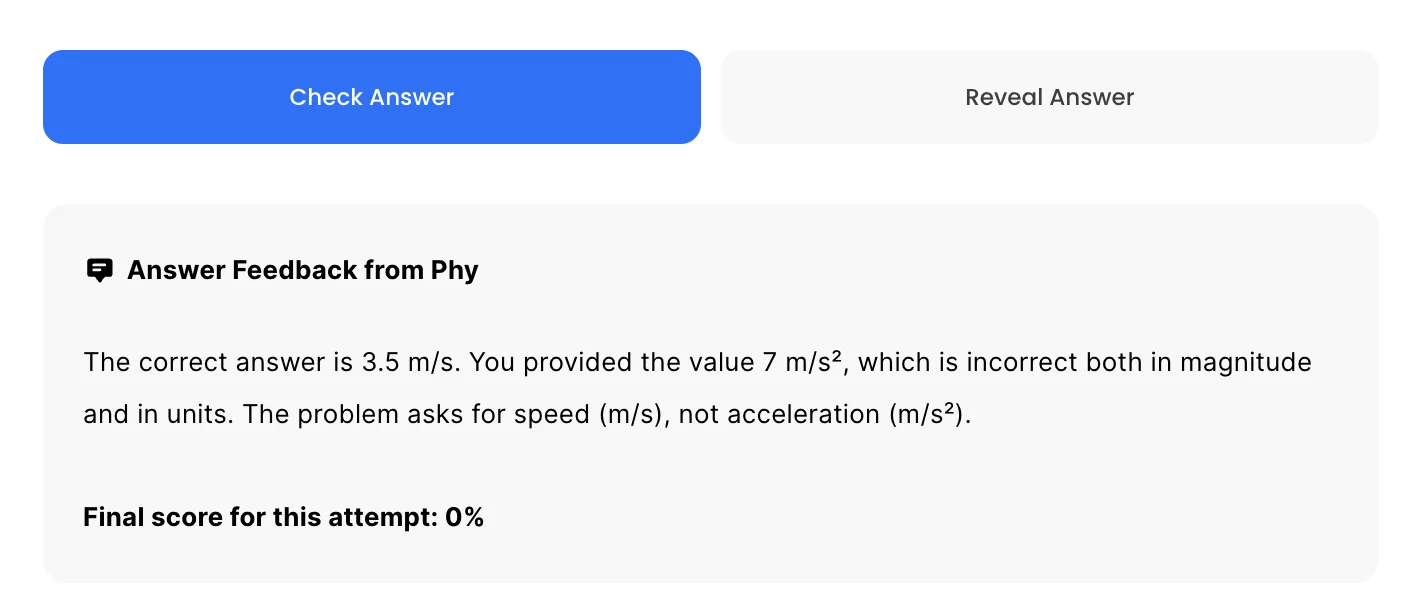
Phy automatically provides feedback so you can improve your responses.Â
10 Free Credits To Get You StartedÂ
By continuing you agree to nerd-notes.com Terms of Service, Privacy Policy, and our usage of user data.Â
