# Part (a): Solving Using Conservation of Energy
| Step | Derivation/Formula | Reasoning |
|---|---|---|
| 1 | U_i + K_i = U_f + K_f | The total mechanical energy (kinetic + potential) at the initial state equals the total mechanical energy at the final state due to conservation of energy. |
| 2 | U_i = m_g \cdot g \cdot h | Initial potential energy is due to m_g being at a height h from the ground. m_y is on the ground, so its potential energy is zero. |
| 3 | U_i = 38 \, \text{kg} \cdot 9.8 \, \text{m/s}^2 \cdot 2.5 \, \text{m} | Calculate the numerical value of U_i . g (acceleration due to gravity) is 9.8 m/s² and h is 2.5 m. |
| 4 | U_i = 931 \, \text{J} | Multiplying the values provides the initial potential energy. |
| 5 | K_i = 0 | Initially, the system is at rest so the initial kinetic energy is zero. |
| 6 | K_f = \frac{1}{2}(m_y + m_g + m_p) v^2 | Final kinetic energy calculated as the sum of the kinetic energies of m_g , m_y and the pulley. m_p is the mass of the pulley. The pulley contributes translational kinetic energy due to its mass. |
| 7 | U_f = 0 | At the final state, m_g is on the ground, so its potential energy is zero. |
| 8 | 931 \, \text{J} = \frac{1}{2} (32 \, \text{kg} + 38 \, \text{kg} + 3.1 \, \text{kg}) v^2 | Use U_i + K_i = U_f + K_f and solve for v . |
| 9 | v = \sqrt{\frac{2 \times 931 \, \text{J}}{73.1 \, \text{kg}}} | Simplify the equation to solve for v . |
| 10 | v = \sqrt{25.48 \, \text{m}^2/\text{s}^2} | Calculate the value under the square root. |
| 11 | v = 5.05 \, \text{m/s} | The final velocity of m_g just before it strikes the ground. |
# Part (b): Solving Using Forces, Torque, and Kinematics
| Step | Derivation/Formula | Reasoning |
|---|---|---|
| 1 | F = m \cdot a | Newton’s second law, the net force applied on the system causes acceleration. |
| 2 | F_{my} – F_{mg} = (m_y + m_g + m_p) \cdot a | Forces due to weights of m_y and m_g , including the pulley’s inertia, where F_{my} = m_y \cdot g and F_{mg} = m_g \cdot g . |
| 3 | T = \frac{m_g g – m_y g}{1 + \frac{m_p}{m_y + m_g}} | Balance the forces taking into account the pulley rotation effect, where T is the net torque and m_p is moment of inertia derived as \frac{1}{2} m_p R^2 assuming a uniform cylinder. |
| 4 | a = \frac{m_g g – m_y g}{m_y + m_g + \frac{1}{2} m_p} | Solving the earlier equation for a , the acceleration of the system, where \frac{1}{2} m_p comes from dividing the pulley’s moment of inertia by R^2 . |
| 5 | v_f^2 = 2 \cdot a \cdot 2.5 \, \text{m} | Use kinematic equation for final velocity, incorporating the calculated acceleration and distance fallen, which is 2.5 m. |
| 6 | v_f = \sqrt{2 \cdot a \cdot 2.5 \, \text{m}} | Solve for final velocity using the value for a . |
| 7 | Approximate numerical calculation similar to Part (a) | The velocity is essentially the same result as obtained from energy conservation, demonstrating the physics consistency. |
Phy can also check your working. Just snap a picture!
A meter stick of mass 200 grams is balanced at the 40-cm mark when a 100-gram mass is suspended from the 10-cm mark. What is the distance from the pivot point to the center of mass of the meter stick? Give your answer in centimeters.
Two equal-magnitude forces are applied to a door at the doorknob. The first force is applied perpendicular to the door, and the second force is applied at 30° to the plane of the door. Which force exerts the greater torque about the door hinge?
A centrifuge rotor rotating at 9200 rpm is shut off and is eventually brought uniformly to rest by a frictional torque of 1.20 N·m. If the mass of the rotor is 3.10 kg and it can be approximated as a solid cylinder of radius 0.0710 m, through how many revolutions will the rotor turn before coming to rest? The moment of inertia of a cylinder is give by \frac{1}{2}mr^2 .
Consider a solid uniform sphere of radius R and mass M rolling without slipping. Which form of its kinetic energy is larger, translational or rotational?
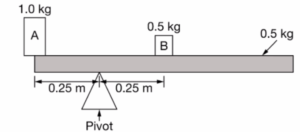
A meter stick with a uniformly distributed mass of 0.5 kg is supported by a pivot placed at the 0.25 m mark from the left. At the left end, a small object of mass 1.0 kg is placed at the zero mark, and a second small object of mass 0.5 kg is placed at the 0.5 m mark. The meter stick is supported so that it remains horizontal, and then it is released from rest. Find the change in the angular momentum of the meter stick, one second after it is released,.
By continuing you (1) agree to our Terms of Sale and Terms of Use and (2) consent to sharing your IP and browser information used by this site’s security protocols as outlined in our Privacy Policy.
| Kinematics | Forces |
|---|---|
| \Delta x = v_i t + \frac{1}{2} at^2 | F = ma |
| v = v_i + at | F_g = \frac{G m_1m_2}{r^2} |
| a = \frac{\Delta v}{\Delta t} | f = \mu N |
| R = \frac{v_i^2 \sin(2\theta)}{g} |
| Circular Motion | Energy |
|---|---|
| F_c = \frac{mv^2}{r} | KE = \frac{1}{2} mv^2 |
| a_c = \frac{v^2}{r} | PE = mgh |
| KE_i + PE_i = KE_f + PE_f |
| Momentum | Torque and Rotations |
|---|---|
| p = m v | \tau = r \cdot F \cdot \sin(\theta) |
| J = \Delta p | I = \sum mr^2 |
| p_i = p_f | L = I \cdot \omega |
| Simple Harmonic Motion |
|---|
| F = -k x |
| T = 2\pi \sqrt{\frac{l}{g}} |
| T = 2\pi \sqrt{\frac{m}{k}} |
| Constant | Description |
|---|---|
| g | Acceleration due to gravity, typically 9.8 , \text{m/s}^2 on Earth’s surface |
| G | Universal Gravitational Constant, 6.674 \times 10^{-11} , \text{N} \cdot \text{m}^2/\text{kg}^2 |
| \mu_k and \mu_s | Coefficients of kinetic (\mu_k) and static (\mu_s) friction, dimensionless. Static friction (\mu_s) is usually greater than kinetic friction (\mu_k) as it resists the start of motion. |
| k | Spring constant, in \text{N/m} |
| M_E = 5.972 \times 10^{24} , \text{kg} | Mass of the Earth |
| M_M = 7.348 \times 10^{22} , \text{kg} | Mass of the Moon |
| M_M = 1.989 \times 10^{30} , \text{kg} | Mass of the Sun |
| Variable | SI Unit |
|---|---|
| s (Displacement) | \text{meters (m)} |
| v (Velocity) | \text{meters per second (m/s)} |
| a (Acceleration) | \text{meters per second squared (m/s}^2\text{)} |
| t (Time) | \text{seconds (s)} |
| m (Mass) | \text{kilograms (kg)} |
| Variable | Derived SI Unit |
|---|---|
| F (Force) | \text{newtons (N)} |
| E, PE, KE (Energy, Potential Energy, Kinetic Energy) | \text{joules (J)} |
| P (Power) | \text{watts (W)} |
| p (Momentum) | \text{kilogram meters per second (kgm/s)} |
| \omega (Angular Velocity) | \text{radians per second (rad/s)} |
| \tau (Torque) | \text{newton meters (Nm)} |
| I (Moment of Inertia) | \text{kilogram meter squared (kgm}^2\text{)} |
| f (Frequency) | \text{hertz (Hz)} |
General Metric Conversion Chart
Example of using unit analysis: Convert 5 kilometers to millimeters.
Start with the given measurement: \text{5 km}
Use the conversion factors for kilometers to meters and meters to millimeters: \text{5 km} \times \frac{10^3 \, \text{m}}{1 \, \text{km}} \times \frac{10^3 \, \text{mm}}{1 \, \text{m}}
Perform the multiplication: \text{5 km} \times \frac{10^3 \, \text{m}}{1 \, \text{km}} \times \frac{10^3 \, \text{mm}}{1 \, \text{m}} = 5 \times 10^3 \times 10^3 \, \text{mm}
Simplify to get the final answer: \boxed{5 \times 10^6 \, \text{mm}}
Prefix | Symbol | Power of Ten | Equivalent |
|---|---|---|---|
Pico- | p | 10^{-12} | 0.000000000001 |
Nano- | n | 10^{-9} | 0.000000001 |
Micro- | µ | 10^{-6} | 0.000001 |
Milli- | m | 10^{-3} | 0.001 |
Centi- | c | 10^{-2} | 0.01 |
Deci- | d | 10^{-1} | 0.1 |
(Base unit) | – | 10^{0} | 1 |
Deca- or Deka- | da | 10^{1} | 10 |
Hecto- | h | 10^{2} | 100 |
Kilo- | k | 10^{3} | 1,000 |
Mega- | M | 10^{6} | 1,000,000 |
Giga- | G | 10^{9} | 1,000,000,000 |
Tera- | T | 10^{12} | 1,000,000,000,000 |
The most advanced version of Phy. Currently 50% off, for early supporters.
per month
Billed Monthly. Cancel Anytime.
Trial –> Phy Pro
A quick explanation
UBQ credits are specifically used to grade your FRQs and GQs.
You can still view questions and see answers without credits.
Submitting an answer counts as 1 attempt.
Seeing answer or explanation counts as a failed attempt.
Lastly, check your average score, across every attempt, in the top left.
MCQs are 1 point each. GQs are 1 point. FRQs will state points for each part.
Phy can give partial credit for GQs & FRQs.
Phy sees everything.
It customizes responses, explanations, and feedback based on what you struggle with. Try your best on every question!
Understand you mistakes quicker.
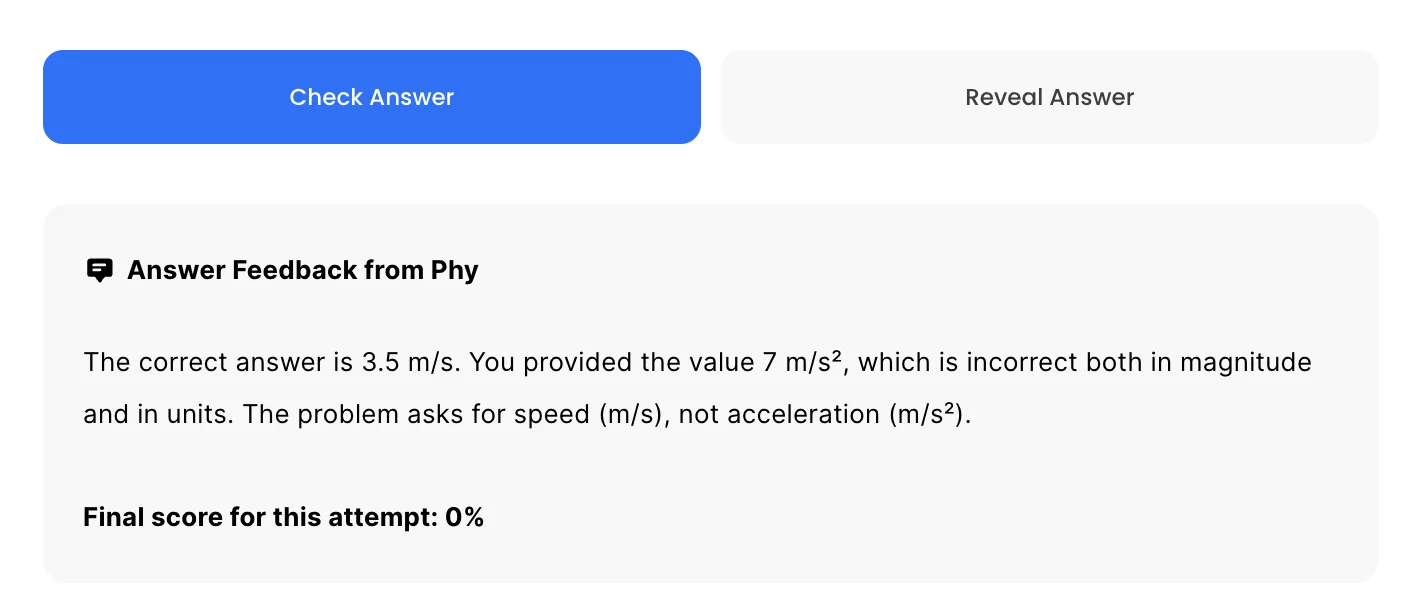
For GQs and FRQs, Phy provides brief feedback as to how you can improve your answer.
Aim to increase your understadning and average score with every attempt!
10 Free Credits To Get You Started
*Phy Pro members get unlimited credits
By continuing you agree to nerd-notes.com Terms of Service, Privacy Policy, and our usage of user data.