Login

AP Physics

Unit 1 - Vectors and Kinematics

10697 views

Advanced

Proportional Analysis

GQ

You're a Phy Pro Member

Supercharge UBQ with

Phy Pro

0 attempts

0% avg

UBQ Credits

Verfied Answer
Verfied Explanation 0 likes
Written by Phy
0

Projectile motion range is derived as follows:

  1. Express the horizontal and vertical components of the initial velocity.
  2. Determine the time of flight based on vertical motion.
  3. Calculate the horizontal distance traveled in this time.
Step Formula Derivation Reasoning
1.1 [katex]v_{x} = v \cos(\theta)[/katex] Horizontal component of initial velocity, constant since no air resistance.
1.2 [katex]v_{y0} = v \sin(\theta)[/katex] Initial vertical component of the velocity.
2.1 [katex]t = \frac{2v_{y0}}{g}[/katex] Time of flight, derived from [katex]v_{y} = v_{y0} – gt[/katex] and the fact that at maximum height, [katex]v_{y} = 0[/katex], hence [katex]t = \frac{2v_{y0}}{g}[/katex] for a round trip.
2.2 [katex]t = \frac{2v \sin(\theta)}{g}[/katex] Substitute [katex]v_{y0} = v \sin(\theta)[/katex].
3.1 [katex]R = v_{x} t[/katex] Range is the horizontal distance traveled.
3.2 [katex]R = v \cos(\theta) \times \frac{2v \sin(\theta)}{g}[/katex] Substitute [katex]v_{x} = v \cos(\theta)[/katex] and [katex]t[/katex] from step 2.2.
3.3 [katex]R = \frac{v^2 \sin(2\theta)}{g}[/katex] Simplify using trigonometric identity [katex]\sin(2\theta) = 2 \sin(\theta) \cos(\theta)[/katex].

Now we apply this to find the new range when the launch speed is doubled.

Step Formula Derivation Reasoning
4.1 [katex]R’ = \frac{(2v)^2 \sin(2\theta)}{g}[/katex] New range formula with doubled speed [katex]2v[/katex].
4.2 [katex]R’ = 4 \times \frac{v^2 \sin(2\theta)}{g}[/katex] Simplify to show the relationship with the original range.
4.3 [katex]R’ = 4R[/katex] Substitute the original range [katex]R[/katex].

Given the original range of 23 m, we will calculate the new range.

The new range, calculated from the derived formula with the launch speed doubled, is [katex]92.0 , \text{m}[/katex].

Need Help? Ask Phy To Explain This Problem

Phy can also check your working. Just snap a picture!

Phy Chat
Just Drag and Drop!
Quick Actions ?
Phy Version 10 (8.25.24) - Systems Operational

Topics in this question

1-to-1 Elite Tutoring

  • Statistics

See how Others Did on this question | Coming Soon

Intermediate
Mathematical
GQ

A golfer hits a shot to a green that is elevated 2.80 m above the point where the ball is struck. The ball leaves the club at a speed of 18.9 m/s at an angle of 52.0° above the horizontal. It rises to its maximum height and then falls down to the green. Ignoring air resistance, find the speed of the ball just before it lands.

  • Projectiles
Full Question
Advanced
Mathematical
FRQ

You kick a soccer ball with an initial velocity directed 53° above the horizontal. The ball lands on a roof 7.2 m high. The wall of the building is 25 m away, and it takes the ball 2.1 seconds to pass directly over the wall.

  • Projectiles
Full Question
Intermediate
Mathematical
GQ

A major-league pitcher can throw a baseball in excess of 41.0 m/s. If a ball is thrown horizontally at this speed, how much will it drop by the time it reaches a catcher who is 17.0 m away from the point of release?

  • Projectiles
Full Question
Advanced
Proportional Analysis
FRQ

On a distant planet, golf is just as popular as it is on earth. A golfer tees off and drives the ball 3.5 times as far as he would have on earth, given the same initial velocities on both planets. The ball is launched at a speed of 45 m/s at an angle of 29° above the horizontal. When the ball lands, it is at the same level as the tee. On the distant planet find:

  • Projectiles
Full Question
Intermediate
Mathematical
FRQ

A baseball is thrown at an angle of 25° relative to the ground at a speed of 23.0 m/s. The ball is caught 42.0 m from the thrower.

  • Projectiles
Full Question
Discussion Threads
Login to Discuss

Leave a Reply

92

Nerd Notes

Discover the world's best Physics resources

Continue with

By continuing you (1) agree to our Terms of Sale and Terms of Use and (2) consent to sharing your IP and browser information used by this site’s security protocols as outlined in our Privacy Policy.

Error Report

Sign in before submitting feedback.

Sign In

Sign In to View Your Questions

Sign In

Share This Question

Enjoying UBQ? Share the 🔗 with friends!

Link Copied!
Made By Nerd-Notes.com
Dark Mode Equation Sheet (Download)
KinematicsForces
[katex]\Delta x = v_i t + \frac{1}{2} at^2[/katex][katex]F = ma[/katex]
[katex]v = v_i + at[/katex][katex]F_g = \frac{G m_1m_2}{r^2}[/katex]
[katex]a = \frac{\Delta v}{\Delta t}[/katex][katex]f = \mu N[/katex]
[katex]R = \frac{v_i^2 \sin(2\theta)}{g}[/katex] 
Circular MotionEnergy
[katex]F_c = \frac{mv^2}{r}[/katex][katex]KE = \frac{1}{2} mv^2[/katex]
[katex]a_c = \frac{v^2}{r}[/katex][katex]PE = mgh[/katex]
 [katex]KE_i + PE_i = KE_f + PE_f[/katex]
MomentumTorque and Rotations
[katex]p = m v[/katex][katex]\tau = r \cdot F \cdot \sin(\theta)[/katex]
[katex]J = \Delta p[/katex][katex]I = \sum mr^2[/katex]
[katex]p_i = p_f[/katex][katex]L = I \cdot \omega[/katex]
Simple Harmonic Motion
[katex]F = -k x[/katex]
[katex]T = 2\pi \sqrt{\frac{l}{g}}[/katex]
[katex]T = 2\pi \sqrt{\frac{m}{k}}[/katex]
ConstantDescription
[katex]g[/katex]Acceleration due to gravity, typically [katex]9.8 , \text{m/s}^2[/katex] on Earth’s surface
[katex]G[/katex]Universal Gravitational Constant, [katex]6.674 \times 10^{-11} , \text{N} \cdot \text{m}^2/\text{kg}^2[/katex]
[katex]\mu_k[/katex] and [katex]\mu_s[/katex]Coefficients of kinetic ([katex]\mu_k[/katex]) and static ([katex]\mu_s[/katex]) friction, dimensionless. Static friction ([katex]\mu_s[/katex]) is usually greater than kinetic friction ([katex]\mu_k[/katex]) as it resists the start of motion.
[katex]k[/katex]Spring constant, in [katex]\text{N/m}[/katex]
[katex] M_E = 5.972 \times 10^{24} , \text{kg} [/katex]Mass of the Earth
[katex] M_M = 7.348 \times 10^{22} , \text{kg} [/katex]Mass of the Moon
[katex] M_M = 1.989 \times 10^{30} , \text{kg} [/katex]Mass of the Sun
VariableSI Unit
[katex]s[/katex] (Displacement)[katex]\text{meters (m)}[/katex]
[katex]v[/katex] (Velocity)[katex]\text{meters per second (m/s)}[/katex]
[katex]a[/katex] (Acceleration)[katex]\text{meters per second squared (m/s}^2\text{)}[/katex]
[katex]t[/katex] (Time)[katex]\text{seconds (s)}[/katex]
[katex]m[/katex] (Mass)[katex]\text{kilograms (kg)}[/katex]
VariableDerived SI Unit
[katex]F[/katex] (Force)[katex]\text{newtons (N)}[/katex]
[katex]E[/katex], [katex]PE[/katex], [katex]KE[/katex] (Energy, Potential Energy, Kinetic Energy)[katex]\text{joules (J)}[/katex]
[katex]P[/katex] (Power)[katex]\text{watts (W)}[/katex]
[katex]p[/katex] (Momentum)[katex]\text{kilogram meters per second (kgm/s)}[/katex]
[katex]\omega[/katex] (Angular Velocity)[katex]\text{radians per second (rad/s)}[/katex]
[katex]\tau[/katex] (Torque)[katex]\text{newton meters (Nm)}[/katex]
[katex]I[/katex] (Moment of Inertia)[katex]\text{kilogram meter squared (kgm}^2\text{)}[/katex]
[katex]f[/katex] (Frequency)[katex]\text{hertz (Hz)}[/katex]

General Metric Conversion Chart

Example of using unit analysis: Convert 5 kilometers to millimeters. 

  1. Start with the given measurement: [katex]\text{5 km}[/katex]

  2. Use the conversion factors for kilometers to meters and meters to millimeters: [katex]\text{5 km} \times \frac{10^3 \, \text{m}}{1 \, \text{km}} \times \frac{10^3 \, \text{mm}}{1 \, \text{m}}[/katex]

  3. Perform the multiplication: [katex]\text{5 km} \times \frac{10^3 \, \text{m}}{1 \, \text{km}} \times \frac{10^3 \, \text{mm}}{1 \, \text{m}} = 5 \times 10^3 \times 10^3 \, \text{mm}[/katex]

  4. Simplify to get the final answer: [katex]\boxed{5 \times 10^6 \, \text{mm}}[/katex]

Prefix

Symbol

Power of Ten

Equivalent

Pico-

p

[katex]10^{-12}[/katex]

Nano-

n

[katex]10^{-9}[/katex]

Micro-

µ

[katex]10^{-6}[/katex]

Milli-

m

[katex]10^{-3}[/katex]

Centi-

c

[katex]10^{-2}[/katex]

Deci-

d

[katex]10^{-1}[/katex]

(Base unit)

–

[katex]10^{0}[/katex]

Deca- or Deka-

da

[katex]10^{1}[/katex]

Hecto-

h

[katex]10^{2}[/katex]

Kilo-

k

[katex]10^{3}[/katex]

Mega-

M

[katex]10^{6}[/katex]

Giga-

G

[katex]10^{9}[/katex]

Tera-

T

[katex]10^{12}[/katex]

  1. Some answers may be slightly off by 1% depending on rounding, etc.
  2. Answers will use different values of gravity. Some answers use 9.81 m/s2, and other 10 m/s2 for calculations.
  3. Variables are sometimes written differently from class to class. For example, sometime initial velocity [katex] v_i [/katex] is written as [katex] u [/katex]; sometimes [katex] \Delta x [/katex] is written as [katex] s [/katex].
  4. Bookmark questions that you can’t solve so you can come back to them later. 
  5. Always get help if you can’t figure out a problem. The sooner you can get it cleared up the better chances of you not getting it wrong on a test!

Phy Pro

The most advanced version of Phy. 50% off, for early supporters. Prices increase soon.

$11.99

per month

Subscribe

Billed Monthly. Cancel Anytime.

Trial  –>  Phy Pro

  • Unlimited Messages and Images
  • Unlimited UBQ Credits
  • 157% Better than GPT
  • 30 --> 300 Word Input
  • 3 --> 15 MB Image Size Limit
  • 1 --> 3 Images per Message
  • All Smart Actions
  • Mobile Snaps
  • Focus Mode
  • No Ads

You can close this ad in 5 seconds.

Ads show frequently. Upgrade to Phy Pro to remove ads.

You can close this ad in 7 seconds.

Ads display every few minutes. Upgrade to Phy Pro to remove ads.

You can close this ad in 5 seconds.

Ads show frequently. Upgrade to Phy Pro to remove ads.

How to UBQ

A quick explanation

1. UBQ Credits

UBQ credits are specifically used to grade your FRQs and GQs.

You can still view questions and see answers without credits.

2. UBQ Stats

Submitting an answer counts as 1 attempt. 

Seeing answer or explanation counts as a failed attempt.

Lastly, check your average score, across every attempt, in the top left. 

3. Point System

MCQs are 1 point each. GQs are 1 point. FRQs will state points for each part.

Phy can give partial credit for GQs & FRQs.

Your learning experience, set by your pace

4. Take your time!

Phy sees everything.

It customizes responses, explanations, and feedback based on what you struggle with. Try your best on every question!

Phy gives feedback.

Understand you mistakes quicker.

4. Feedback

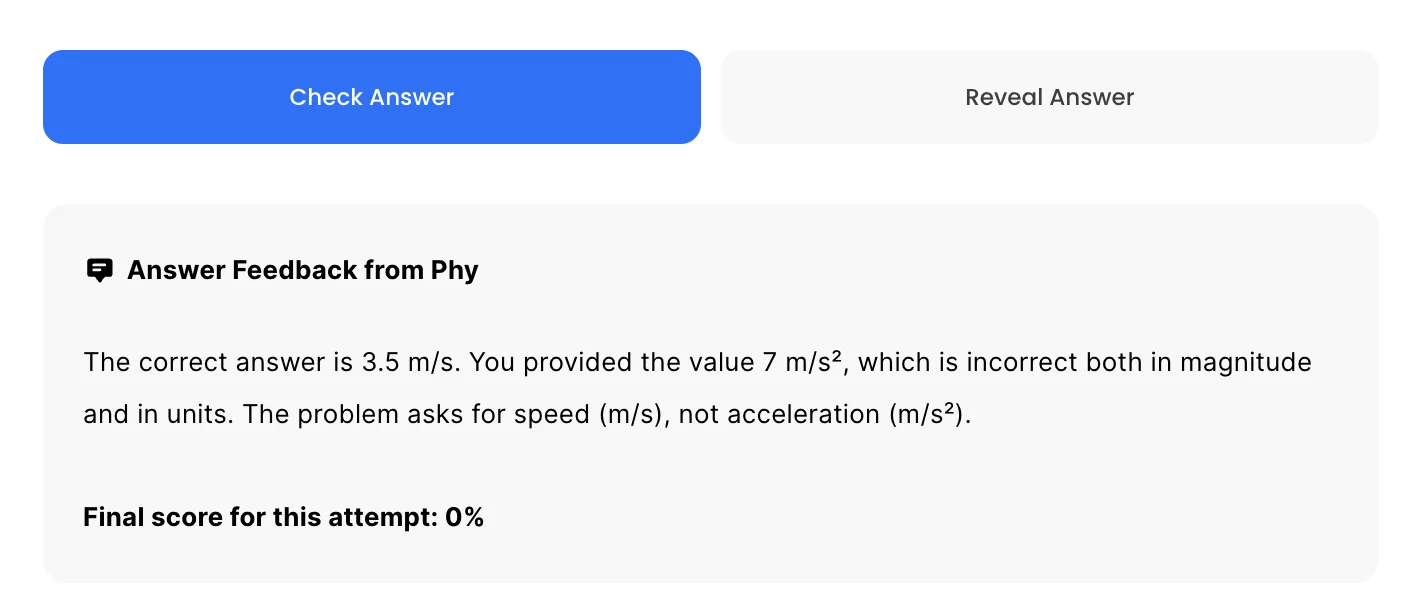
For GQs and FRQs, Phy provides brief feedback as to how you can improve your answer. 

Aim to increase your understadning and average score with every attempt!

10 Free Credits To Get You Started 

*Phy Pro members get unlimited credits

Start Solving

By continuing you agree to nerd-notes.com Terms of Service, Privacy Policy, and our usage of user data. 

Jason here! Feeling uneasy about your next physics test? We will help boost your grade in just two hours.

Book a Free Lesson

NEW! PHY instantly solves any question

Try Now

NEW! Elite Members get 40% off Physics Tutoring

Join Now
We use site cookies to improve your experience. By continuing to browse on this website, you accept the use of cookies as outlined in our privacy policy.
I Accept