The best way to analyze this situation is to apply the principle of conservation of momentum and understand how momentum changes for individual bodies in a collision. Momentum, defined as the product of mass and velocity ( p = mv ), is always conserved in an isolated system (without external forces).
Given:
– Let v_t and v_c be the velocities of the truck and the car respectively.
– Assume the truck has a mass m_t and the car has a mass m_c .
– Truck’s initial velocity v_{t, \text{init}} = 15 , \text{km/h} (we’ll consider the direction towards the car as positive).
– Car’s initial velocity v_{c, \text{init}} = -30 , \text{km/h} (since it’s head-on and opposite, it’s negative).
Step 1: Calculate Initial Momenta
– Momentum of the truck initially: p_{t, \text{init}} = m_t \times 15
– Momentum of the car initially: p_{c, \text{init}} = m_c \times (-30)
Step 2: Use Conservation of Momentum
– Total initial momentum p_{\text{total, init}} = m_t \times 15 + m_c \times (-30) .
The collision is an isolated event with no external forces, so total momentum must be preserved:
– p_{\text{total, final}} = p_{\text{total, init}} .
After the collision, depending on the details (elastic or inelastic), both truck and car will have new velocities v_{t, \text{final}} and v_{c, \text{final}} but their combined momentum remains m_t \times v_{t, \text{final}} + m_c \times v_{c, \text{final}} = m_t \times 15 + m_c \times (-30) .
Step 3: Change in Momentum
– Change in momentum for the truck: \Delta p_t = m_t \times (v_{t, \text{final}} – 15)
– Change in momentum for the car: \Delta p_c = m_c \times (v_{c, \text{final}} + 30)
Change in the magnitude of the individual momenta will depend on the masses of the truck and car and their change in velocities but must reflect conservation principles.
Answer Analysis:
– (a) Incorrect – A greater mass does not directly mean a greater change in momentum unless the change in velocity is considered.
– (b) Correct – Since momentum is conserved and the system is isolated, both the car and truck experience the equal magnitude of momentum change but in opposite directions.
– (c) Incorrect – Greater initial speed of the car implies greater initial momentum, but not necessarily a greater change in momentum.
– (d) Incorrect – Both vehicles change their momentum, but the total system momentum is conserved.
– (e) Incorrect – Statement (b) is necessarily true considering the law of conservation of momentum in an isolated system.
Thus, the statement that best describes the situation is (b) They both have the same change in magnitude of momentum because momentum is conserved.
Phy can also check your working. Just snap a picture!
A bowling ball moving with speed v collides head-on with a stationary tennis ball. The collision is elastic and there is no friction. The bowling ball barely slows down. What is the speed of the tennis ball after the collision?
A karate master is about to split a piece of wood with her hand. Select all she must do in order to deliver the maximum force to split the wood.
A 0.0350 kg bullet moving horizontally at 425 m/s embeds itself into an initially stationary 0.550 kg block.
A 15 g marble moves to the right at 3.5 m/s and makes an elastic head-on collision with a 22-g marble. The final velocity of the 22 g marble is 2.0 m/s to the right, and the final velocity of the 15 g marble is 5.4 m/s to the left. What was the initial velocity of the 22 g marble?
A 4 kg mass is traveling at 10 m/s to the right when it collides elastically with a stationary 7 kg mass. The 7 kg mass then travels at 2 m/s at an angle of 22° below the horizontal. What is the velocity of the 4 kg mass?
By continuing you (1) agree to our Terms of Sale and Terms of Use and (2) consent to sharing your IP and browser information used by this site’s security protocols as outlined in our Privacy Policy.
| Kinematics | Forces |
|---|---|
| \Delta x = v_i t + \frac{1}{2} at^2 | F = ma |
| v = v_i + at | F_g = \frac{G m_1m_2}{r^2} |
| a = \frac{\Delta v}{\Delta t} | f = \mu N |
| R = \frac{v_i^2 \sin(2\theta)}{g} |
| Circular Motion | Energy |
|---|---|
| F_c = \frac{mv^2}{r} | KE = \frac{1}{2} mv^2 |
| a_c = \frac{v^2}{r} | PE = mgh |
| KE_i + PE_i = KE_f + PE_f |
| Momentum | Torque and Rotations |
|---|---|
| p = m v | \tau = r \cdot F \cdot \sin(\theta) |
| J = \Delta p | I = \sum mr^2 |
| p_i = p_f | L = I \cdot \omega |
| Simple Harmonic Motion |
|---|
| F = -k x |
| T = 2\pi \sqrt{\frac{l}{g}} |
| T = 2\pi \sqrt{\frac{m}{k}} |
| Constant | Description |
|---|---|
| g | Acceleration due to gravity, typically 9.8 , \text{m/s}^2 on Earth’s surface |
| G | Universal Gravitational Constant, 6.674 \times 10^{-11} , \text{N} \cdot \text{m}^2/\text{kg}^2 |
| \mu_k and \mu_s | Coefficients of kinetic (\mu_k) and static (\mu_s) friction, dimensionless. Static friction (\mu_s) is usually greater than kinetic friction (\mu_k) as it resists the start of motion. |
| k | Spring constant, in \text{N/m} |
| M_E = 5.972 \times 10^{24} , \text{kg} | Mass of the Earth |
| M_M = 7.348 \times 10^{22} , \text{kg} | Mass of the Moon |
| M_M = 1.989 \times 10^{30} , \text{kg} | Mass of the Sun |
| Variable | SI Unit |
|---|---|
| s (Displacement) | \text{meters (m)} |
| v (Velocity) | \text{meters per second (m/s)} |
| a (Acceleration) | \text{meters per second squared (m/s}^2\text{)} |
| t (Time) | \text{seconds (s)} |
| m (Mass) | \text{kilograms (kg)} |
| Variable | Derived SI Unit |
|---|---|
| F (Force) | \text{newtons (N)} |
| E, PE, KE (Energy, Potential Energy, Kinetic Energy) | \text{joules (J)} |
| P (Power) | \text{watts (W)} |
| p (Momentum) | \text{kilogram meters per second (kgm/s)} |
| \omega (Angular Velocity) | \text{radians per second (rad/s)} |
| \tau (Torque) | \text{newton meters (Nm)} |
| I (Moment of Inertia) | \text{kilogram meter squared (kgm}^2\text{)} |
| f (Frequency) | \text{hertz (Hz)} |
General Metric Conversion Chart
Example of using unit analysis: Convert 5 kilometers to millimeters.
Start with the given measurement: \text{5 km}
Use the conversion factors for kilometers to meters and meters to millimeters: \text{5 km} \times \frac{10^3 \, \text{m}}{1 \, \text{km}} \times \frac{10^3 \, \text{mm}}{1 \, \text{m}}
Perform the multiplication: \text{5 km} \times \frac{10^3 \, \text{m}}{1 \, \text{km}} \times \frac{10^3 \, \text{mm}}{1 \, \text{m}} = 5 \times 10^3 \times 10^3 \, \text{mm}
Simplify to get the final answer: \boxed{5 \times 10^6 \, \text{mm}}
Prefix | Symbol | Power of Ten | Equivalent |
|---|---|---|---|
Pico- | p | 10^{-12} | |
Nano- | n | 10^{-9} | |
Micro- | µ | 10^{-6} | |
Milli- | m | 10^{-3} | |
Centi- | c | 10^{-2} | |
Deci- | d | 10^{-1} | |
(Base unit) | – | 10^{0} | |
Deca- or Deka- | da | 10^{1} | |
Hecto- | h | 10^{2} | |
Kilo- | k | 10^{3} | |
Mega- | M | 10^{6} | |
Giga- | G | 10^{9} | |
Tera- | T | 10^{12} |
The most advanced version of Phy. Currently 50% off, for early supporters.
per month
Billed Monthly. Cancel Anytime.
Trial –> Phy Pro
Understand you mistakes quicker.
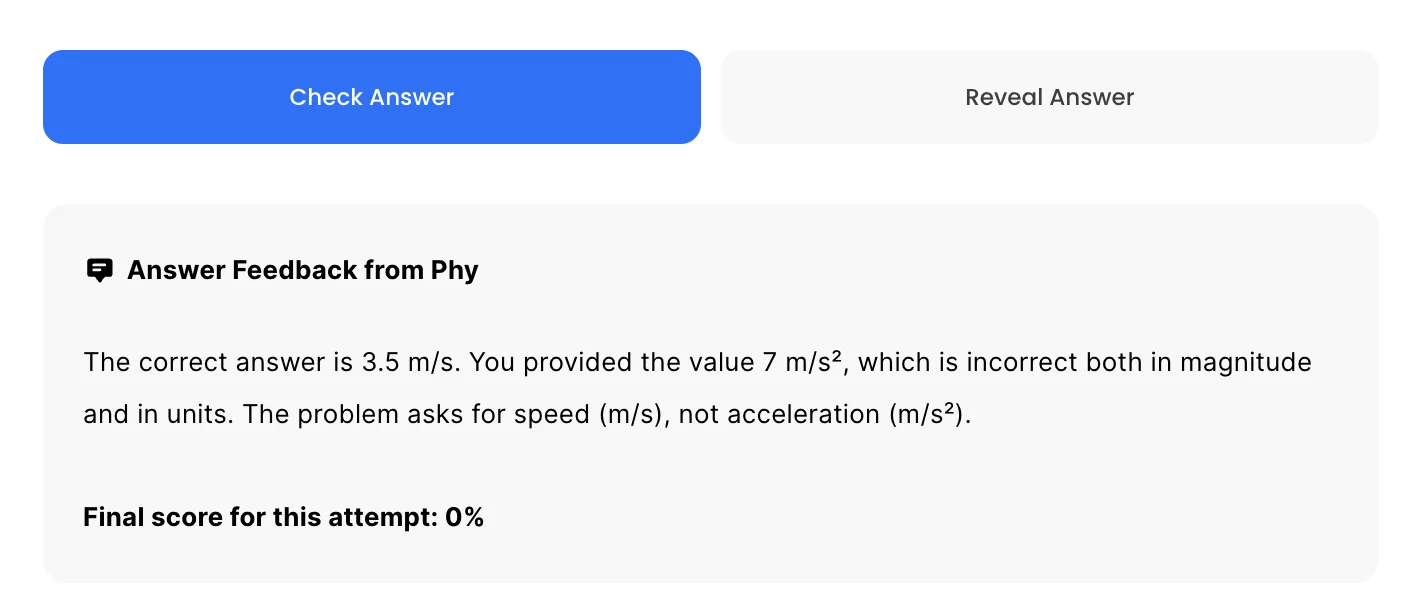
For GQs and FRQs, Phy provides brief feedback as to how you can improve your answer.
Aim to increase your understadning and average score with every attempt!
10 Free Credits To Get You Started
*Phy Pro members get unlimited credits
By continuing you agree to nerd-notes.com Terms of Service, Privacy Policy, and our usage of user data.
